Botanical Fractals
type | RESEARCH // BIOPHILIC DESIGN status | ONGOING year | 2019

The First Fractal Life Forms
We have already established that fractals are very prevalent in large-scale geographies and landscapes, in the previous page about macro-fractals. On this page, we look at organisms in which fractal structures are pervasive at a much smaller scale. Some of the earliest known life-forms to exhibit fractal growth patterns are too old to be classified as "plants".
Some of the first large multicellular organisms to ever exist (during the Ediacaran period, some 570 million years ago), "proto-animals", were colonies of identical or similar cells. These animals had an extremely basic physiology, a form that could be put together with as little as six genetic commands (consider, complex mammals such as humans require about twenty-five thousand genetic commands, via "First Life", a documentary by David Attenborough). This was because they built themselves out of fractals, where colonies assembled themselves by branching out repeatedly in a form similar to bi-perpendicular space-filling curves.
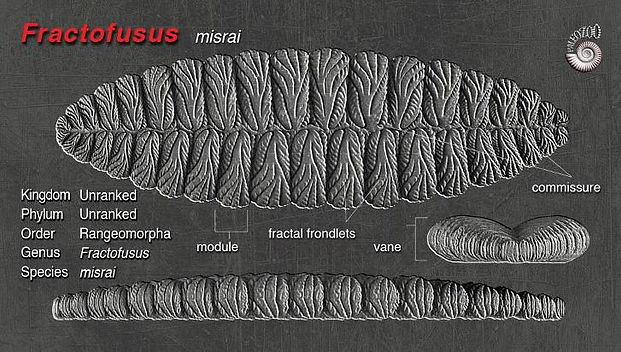
An illustration of Fractofusus misrai, a multicellular life-form found during the Ediacaran period. This illustration has been reconstructed from impressions left in fossils, sourced via https://www.paleozoo.com.au/fractofusus.php
Among fossilised impressions of these creatures, two species are extremely common: Charnia and Fractofusus. Both of these species lived anchored to or lying on the seafloor, far below the depth at which photosynthesis is possible. Since they did not move, they were likely filter feeders, and it is hypothesised that the fractal branching pattern was not just a simple structure for growth, but it also helped increase the surface area to volume ratio. This allowed more dissolved nutrients and minerals to come in contact with the individual cell walls. Due to the simplicity of the form, and a very high rate of asexual propagation via propagules, these rangeomorphs were extremely successful organisms during the late Ediacaran.
Below, I've attached the link for a paper explicating the fractal nature of these rangeomorphs, testing the various hypotheses associated with the advantage of fractal forms in this early life.
Bacteria and Lichen Morphology
The first colonisers of land, both single-celled and multicellular organisms, and colonies of composite organisms exhibit fractal morphology (morphology refers to form, or structure). Studies looking at the morphogenesis of certain gram-negative bacteria species such as Klebsiella pneumoniae and Proteus mirabilis in lab conditions have recorded fractal growth patterns of these colonies.
Similar fractal growth patterns occur in endoliths, colonies that form on the surface of rocks. These macro-colonies, visible to the naked eye as a stain or discolouration, often display rhizomatic growth patterns - in the form of a space-filling curve. By employing fractal morphogenesis, these colonies can branch out several hundred times on the rock till they achieve the highest possible surface area for photosynthesis. An example of an endolithic colony, shown below.

A close-up of an endolithic colony (it is unclear whether this is bacterial, fungal or algal) on sandstone. Here, a fractal branching structure is evident by studying the patterns of darker discolourations around the edges of lighter discolourations. Photographed by Kahin Vasi.
As with Charnia and the other rangeomorphs of the Ediacaran period, we see that fractal forms are regularly employed, even today, to build up colonies of basic single- or multi-celled organisms. For two major reasons: this kind of structure allows the organism or colony to grow quickly with the least number of genetic commands, and it allows the greatest surface area, or number of cells to be exposed to the source of the nutrients.
The link to a paper detailing the observations of fractal growth in bacterial colonies has been provided below.
Another kind of organism, Lichen, (the oldest of which is about 400 million years old) has also adopted fractal morphology at various lefts, depending on the species or typology of the lichen. A lichen is not a single organism, but a community made up of algae or cyanobacteria that live within or in the filaments of various species of fungi. The fungi digest the substrate into which their root systems spread, providing nutrients and minerals for the cyanobacteria and algae, which in turn photosynthesise, creating energy and food for the fungus.
Lichens can be found with various structures; some are spindly and others grow with lobes. Lichens displaying fruticose growth, or a bushy, branching growth are the most obvious fractal forms. Here, a fractal formation is used to create a lightweight structure that exposes the maximum area to light, enough for the cyanobacteria or algae to photosynthesise.
Foliose lichens, on the other hand, have a broader, leaf-like structures (akin to folds of skin) wherein the fractal is less obvious. In these kinds of lichens, the fractal branching form is found not in the actual structure of the lichen, but in the fungal hyphae (that branch out in search for nutrients or organic matter to dissolve). Certain kinds of lichens, very simple ones, consist of colonies of cyanobacteria simply wrapped around the hyphae of a fungal system.

An example of a lichen with a fruticose structure, Wolf Lichen. This is an epiphytic lichen, i.e., it grows on the barks of trees. Photographed by Kahin Vasi

Here are two different kinds of lichen, both fruticose (branching) and foliar (leaf-like) on the bark of a tree. Photographed by Kahin Vasi.

A close-up of foliose lichen on a decaying branch, where the hyphae are branching out towards other sources of nutrients (white tendrils in the lower third of the photograph). Photographed by Kahin Vasi.
Fractal Ferns
Ferns are among the oldest vascular plants found on land, some fossils found dating back to the late Devonian period (360 million years ago). Although most of the extant species of ferns have only been around for 160 million years (well after the advent of seed-bearing and flowering plants), their structural systems have roots in the very first species of ferns, now extinct. These early plants, like the lichen and rangeomorphs before them, use fractals to define their growth structure. Because of the success of the growth patterns employing fractal geometry, these plants have colonised almost every environment on earth.
In the image of a fern frond below, two simple morphologies are visible: symmetry and self-similarity. This is a pinnate-pinnatifid leaf form, i.e., leafs branch out from secondary midribs, known as pinnae. Each pinnae is attached to the midrib, and the midrib in turn is attached to a stem. The fern is using the same geometry, or genetic code to replicate and grow each form with ease. Some species of ferns exhibit a 2-pinnate-pinnatifid frond formation, which would be a higher level of a fractal.

Pteridium aquilinum (L.), a bracken, is a common fern that exhibits a clear fractal geometry. These large, regularly divided leaves unfurl from fronds and grow outwards, and each pinna is similar in structure to the whole frond - and each pinnule, the smallest unit, is similar to the pinna. Photographed by Kahin Vasi.

The Gymnocarpium dryopteris (L.), the common oak fern, in an example of a 2-pinnate leaf structure, wherein we can see the fractal self-similarity between the form of the whole frond, the pinnae, and the pinnule. Photographed by Kahin Vasi.
The fractal structure of ferns is so apparent that mathematicians have created computer- or mathematically-generated fractals that explicate the formation or morphology of fern fronds. The Barnsley Fern is a classic example of a mathematical fractal concept that has been inspired by the structure of a black spleenwort fern, Asplenium adiantum-nigrum.
Leaf Growth and Form
Angiosperms (broadly, flowering plants) are considerably younger, having evolved around 250 million years ago. They became highly prolific about 120 million years ago. These plants, therefore, have a vastly more complex morphology, compared to ferns and lichen. In most such plants, the fractal structure is not obvious, although it is observed in the venation pattern (the visible veins of the surface of a leaf).
In certain plants, such as the Geranium robertanium in the image below, the telltale symmetry and scalar self-similarity of a fractal structure is better defined. Each steam is capped with 3 leaves, each leaf has 3 lobes, each lobe has 3 subdivisions, and each subdivision is made with 3 notches. A distinct pattern of symmetry around three axes and a marked similarity in the structure is not rare - although plants and trees with simple or compound leaves often lack clear adherence to fractal rules.

The leaf of the Geranium robertanium displaying trilateral symmetry and self-similarity over various scales. Photographed by Kahin Vasi.
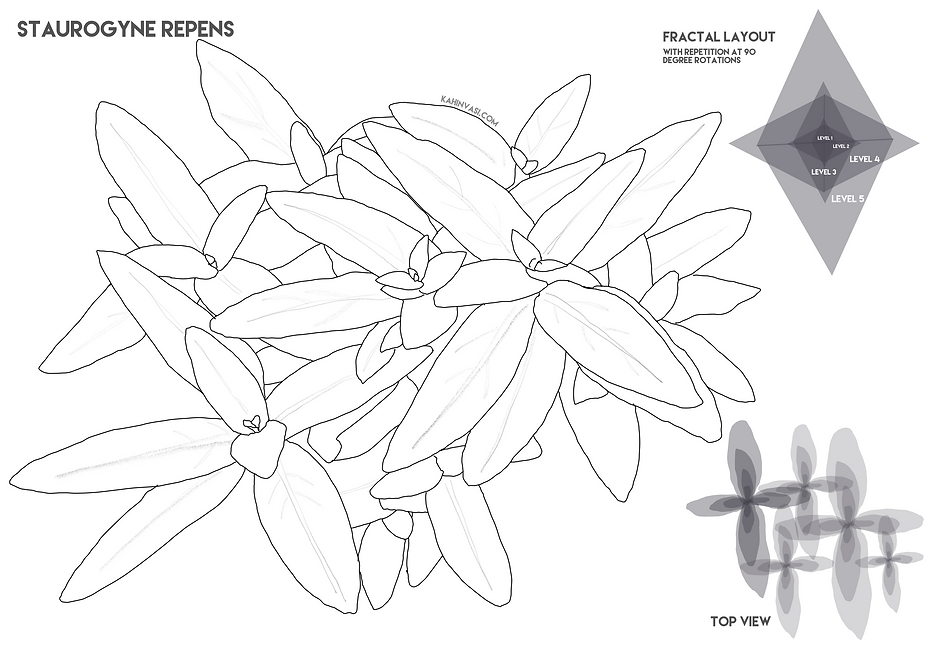
An illustration of a cluster of Staurogyne repens, an aquatic plant native to India, showing the fractal growth pattern exhibited by various Classes of sift stem terrestrial and non-terrestrial plants. In the upper right corner, there is a diagrammatic breakdown of the various levels of the fractal created as new leaves grow out. Illustrated by Kahin Vasi.
Another fractal found in several species of flowering shrubs or macrophytic plants of the Family Acanthaceae is the growth pattern, where leaves grow in pairs at 90 degree rotations from the previous pair of leaves (as seen in the illustration above). This kind of morphology allows the plant to grow rapidly with very little genetic input - a simple rotate/scale/repeat command. This can be seen in the common tropical houseplant, Fittonia albivenis and various other shrubs. The advantage of a simple set of genetic commands is still being used by all kinds of plants and some basic animals to accelerate their growth and build up body mass or complex systems.
Branching Patterns
Fractal morphologies, though widespread in botany, are most commonly associated with branch structure found in large, hard-stemmed trees and shrubs. Although direct self-similarity is not evident, if one were to break the trees branches into individual units, you would find that most often the trunk or stem, the primary branches, the secondary branches, and twigs are all scaled versions of identical typology; what's more is, when assembled by order, they are all iterative, i.e., the smaller or newer iteration will begin at the end of the larger or older iteration.
Interestingly, it has also been mathematically proven that the total volume of wood in all the secondary branches of the tree is equal to the total volume of wood in all the primary branches of the tree, although the primary branches are fewer in number. This repeats, over and over, showing that a fractally growing tree has a second command in addition to self-similarity, repetition and scale: after the tree reaches a particular mass at a particular dimension, the genetic code makes it create a new fractal dimension.

The branch structure of a maple tree, showing several levels of the fractal that builds up branches. Photographed by Kahin Vasi.

The growth pattern of this Mangrove Holly's leaves exhibit a similar scale-rotate fractal function to the Staurogyne illustration above. Here, you can clearly see that each newer pair of leaves is rotated by 90 degrees as it grows out. Photographed by Kahin Vasi
In addition to branching patterns, certain kinds of leaf growth is also defined by a fractal operation - an operation identical to that found in shrubs and macrophytic plants of the Acanthaceae family.
The advantage of having such a growth pattern, whether it is fractal branches or the symmetrical-rotated-growth of leaves, is that the elements of the tree do not accidentally compete with themselves for sunlight. These simple mathematical operations ensure that there is just enough dissimilarity between two fractal dimensions, or a calculated similarity to ensure that the leaves' photoreceptors are constantly partially lit throughout the day.
Bark Formation
The bark of a tree has a particularly curious morphology, as it is in state of constant flux. The bark protects the inner parts of the tree from weathering, pathogenic infestations, and wood-boring insects. It is formed by the outer layer of cells of a plant producing waterproof cork cells, drying out and dying. As the plant grows, the old bark is pushed outwards by new layers of dead cork cells - gradually thickening the layer of bark. However, over a long period of time, the tree experiences wet and dry spells where the trunk expands or contracts, leading to micro-cracks in the bark. This, combined with natural weathering, bark splitting due to ice, and sudden temperature fluctuations leads to major cracks in the bark of a tree. However, upon closer inspection, it becomes apparent that the cracks in the bark are riddled with thousands of fine cracks, creating a texture like the one seen in the image below. It was Benoit Mandelbrot (widely regarded as the father of fractal mathematics) who first stated that the bark of a tree is generated through a series of fractal operations.

Fractal cracking in this lodgepole pine snag has begun to weather away, revealing the layers of bark and the cracks at various fractal dimensions in each layer. Photographed by Kahin Vasi
Fractals caused by fluid or climatic weathering of geography (see the previous page, Macro-Fractals) are not quite the same as the fractals that are created as a part of a living creature's morphology and structure. For one thing, there are several different factors at play - not simply fluid mechanics. Living organisms grow by adding cellular (or in many cases, multicellular) mass to their bodies. In organisms that do not possess a regulatory nervous centre that defines growth, fractal branching patterns are among the easiest growth forms, requiring a very low number of genetic commands to achieve with success.
Fractal morphologies are used to achieve a greater surface area in simple organisms, allowing cell walls to absorb a higher volume of nutrients. This is developed as surface area increasing greatly while mass increases at a considerably lower rate - a realistic instance of the Menger Sponge. This conserves energy and time expended in growth - and allows the organisms to focus on gathering energy and reproduction or proliferation.
In more complex organisms such as ferns and angiosperms, fractal morphology allows quick development of form, unfurling larger surface areas and populating thousands of branches with photosynthesising leaves with remarkably few genetic commands, i.e., creating complex structures with relatively simple code. Another advantage of fractal patterns in leaves is that it ensures that the sunlight is not completely obfuscated by leaves that are higher up - creating porous and multi-planar photosynthesising surfaces, greatly increasing the amount of energy captured by the tree.
An application of the above principle could be in the implementation of solar panels on the top of a structure: instead of making a single plane into a solar surface, 2 or 3 porus planes with solar cells could be used - increasing the possible energy output of the solar array. Fractal patterns are already used in structural frameworks to increase their tensile or compressive strengths while keeping the structure considerably lightweight.
You can see the next page in this series, "Space Fractals", or look at an example of how fractals were applied to a cluster-building housing project under "Generative Microhousing".